 |
| Source: Wisegeek.com |
Many students believe they are inherently bad at math, and they cannot do anything about their supposedly predetermined mathematical abilities. Studies have shown that if a student has that mindset towards their intelligence and abilities, then they are more likely to perform worse in school. In countries ranked higher in math, the notion that someone can be innately inferior at math is rare.
Math curricula in the United States is controlled by the state. Because states compete with each other to provide better education, when one state adds a new topic to their curriculum, other states follow. This leads to a curricula that covers too many topics for a student to handle in one year and to students having a shallow understanding of math. This is due to the limited time in learning the material, so much so that a little over a quarter of students do not have enough math proficiency to be able to do the math required when they become adults.
If you believe that you are "naturally bad at math", then you are more likely to get lower math grades
Some students say, they are simply bad at mathematics and no matter what they do that they just do not understand it. Research has shown that students who believe that they cannot improve their intelligence are more likely to perform poorly in school.
There are two modes of thought on intelligence described by the implicit theory of intelligence. The first mode of thought is that intelligence is fixed, which is known as the entity theory of intelligence. Students who believe that they are naturally bad at math think of intelligence in terms of the entity theory. The second mode of thought is that intelligence is not fixed and can be improved, which is known as the incremental theory of intelligence.
A study showed students who ascribe to the entity theory of intelligence are more likely to have a declining performance or grades that stay relatively constant in school. This result supports the entity theory because if the students don't think they can do anything about their intelligence or grades, they will not do anything to change or improve their study habits. Conversely, students who are a part of the incremental theory of intelligence are more likely to have improving grades over time as shown by the graph.
Wisely Wong, a Professor of Mathematics at the University of Maryland, says the following on why students struggle with mathematics: "People give up too easy. Consider the analogy 'Why can this guy play the piano so well and I can't?' It's because he practices a lot and you don't. Math is a common subject that we all use, if music was the same, then there would be the same complaint. Think about your own talents, you practice so much and that's why you're good."
Students who believe the incremental theory of intelligence get better grades because they are more likely to attribute their errors to themselves. As Professor Wong stated, practice is all that is needed not only for mathematics but also for all subjects. Therefore, a student who believes it is their fault for having bad grades knows that they need to practice more in order to improve their grades.
There are two modes of thought on intelligence described by the implicit theory of intelligence. The first mode of thought is that intelligence is fixed, which is known as the entity theory of intelligence. Students who believe that they are naturally bad at math think of intelligence in terms of the entity theory. The second mode of thought is that intelligence is not fixed and can be improved, which is known as the incremental theory of intelligence.
 |
| Data from: http://bit.ly/1Jh9mvk |
Wisely Wong, a Professor of Mathematics at the University of Maryland, says the following on why students struggle with mathematics: "People give up too easy. Consider the analogy 'Why can this guy play the piano so well and I can't?' It's because he practices a lot and you don't. Math is a common subject that we all use, if music was the same, then there would be the same complaint. Think about your own talents, you practice so much and that's why you're good."
Students who believe the incremental theory of intelligence get better grades because they are more likely to attribute their errors to themselves. As Professor Wong stated, practice is all that is needed not only for mathematics but also for all subjects. Therefore, a student who believes it is their fault for having bad grades knows that they need to practice more in order to improve their grades.
The researchers from the first study also conducted a second study on the implicit theory of intelligence that agrees with some of the statements Professor Gulick made in the interview. The second study found that if students are taught intelligence is malleable, then the effects of believing the entity theory of intelligence is curbed. The declining grades of some of the students from the first study can be stopped. They simply have to believe that they can do better. It is possible for all students to be successful at math if they are taught properly with the right mindset.
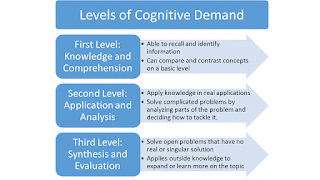
26% of students struggle with synthesis, evaluation, and analysis questions and are unable to apply math to real-world applications
 |
| Data from: http://1.usa.gov/1NxZZNk |
For example, students were strong at reading data from tables, performing simple operations from that data, and utilizing formulas that correspond directly to the data extracted. On the other hand, they were poor at utilizing π, applying math to real world applications, and interpreting the results of real world applications of math.
The first level of cognitive demand involves the basic understanding of the information learned and the recognition and application of the information in a simple manner. Higher levels of cognitive demand require a deeper understanding of the information and the ability to think more abstractly. The highest level involves applying the information to open questions in the world and real world applications. The United States strives for math students to be able to do this in order to allow them to join math-intensive fields such as engineering and to solve the big problems.
The United States strives for students with high math abilities. A major point of concern is that more than a quarter of students at fifteen years of age fall below the second level of the baseline proficiency set out by the OECD and the Program of International Student Assessment (PISA). Being at this level of proficiency means that the student is capable of using mathematics to operate productively in the real world.
Math curricula cover too many topics in a short amount of time and prevent students from thoroughly learning concepts
The standards for mathematics education in the United States is controlled on the state level. The implications of this fact has led to a population of students with a poor conceptual understanding of a variety of topics; however, knowledge of many topics with poor understanding is practically useless as demonstrated by the United States' poor ranking.
Singapore, one of the top countries in Math education, has a well-established mathematics framework developed by their ministry of education with clear goals and steps to reach those goals. In contrast, the United States does not have a strict national standard for mathematics. Furthermore, states provide broad goals with little details on how teachers should guide students towards these goals.
The problem with having states develop their own standards for education is that they begin to compete with each other. Consequently, if one state includes a certain topic in their curriculum, then other states will add the topic into their curriculum as well. This results in a large amount of material that has to be covered. Covering all this material in an academic school year is impossible to teach and learn thoroughly. Students, then, are introduced to a variety of topics, but with limited understanding.
Since so little time is spent on each topic, students seldom have sufficient knowledge or understanding of the material to answer questions of high cognitive demand. Often, they are only able to answer questions of low cognitive demand that involves identifying concepts and simply plugging numbers into equations. Math class becomes nothing more than a teacher introducing a topic and doing some examples, and a student mimicking the teacher and memorizing the equations without understanding the concepts behind the equations. This explains why there are so many students who are unable to apply their mathematics knowledge to real world situations.
While Singapore may have a superior educational model, the United States cannot adopt their model exactly. The United States has a greater focus on preparing mathematics students for applications of math into science and engineering, while Singapore mainly focuses on the mathematics itself. Nevertheless, the United States should take strides to follow some of the general ideas in the Singaporean model.
The United States has been somewhat following the ideas Singapore has, such as a national curriculum including clear goals and steps to reach them. A set of standards designed to prepare students to be competitive once they reach the college level known as the Common Core has already been adopted in 42 states. Common Core still leaves the issue of implementing these standards to the local and state levels to decide.
Singapore, one of the top countries in Math education, has a well-established mathematics framework developed by their ministry of education with clear goals and steps to reach those goals. In contrast, the United States does not have a strict national standard for mathematics. Furthermore, states provide broad goals with little details on how teachers should guide students towards these goals.
 |
| Data from: http://bit.ly/1jIwM6t |
Since so little time is spent on each topic, students seldom have sufficient knowledge or understanding of the material to answer questions of high cognitive demand. Often, they are only able to answer questions of low cognitive demand that involves identifying concepts and simply plugging numbers into equations. Math class becomes nothing more than a teacher introducing a topic and doing some examples, and a student mimicking the teacher and memorizing the equations without understanding the concepts behind the equations. This explains why there are so many students who are unable to apply their mathematics knowledge to real world situations.
While Singapore may have a superior educational model, the United States cannot adopt their model exactly. The United States has a greater focus on preparing mathematics students for applications of math into science and engineering, while Singapore mainly focuses on the mathematics itself. Nevertheless, the United States should take strides to follow some of the general ideas in the Singaporean model.
The United States has been somewhat following the ideas Singapore has, such as a national curriculum including clear goals and steps to reach them. A set of standards designed to prepare students to be competitive once they reach the college level known as the Common Core has already been adopted in 42 states. Common Core still leaves the issue of implementing these standards to the local and state levels to decide.
The United States stands far behind Asian and European countries like Singapore, Hong Kong and Finland in Math and Science rankings
 |
| Data from: http://bit.ly/1lHT2hU |
Professor Gulick explains that the cause of such a low ranking in our country is due to how our society functions in the modern world and how people think about mathematics.
The United States is one of the more developed nations with the highest GDP and relies heavily on science and technology. Despite this fact, the United States does not fare very well in comparison to other countries in the world. In particular, Asian countries – China, Singapore, and Japan – and European countries – Finland, Switzerland, and the Netherlands – dominate the top 10.
The results show not only information on students who struggle with math, but also on the top students in the United States. 2% of students in the United States demonstrate the highest level of mathematics competency. In contrast to the top country in mathematics, 31% of students in Shanghai-China demonstrate the same level of proficiency.
Sources:
http://www.air.org/sites/default/files/downloads/report/Singapore_Report_Bookmark_Version1_0.pdf
http://www.bbc.com/news/business-32608772
https://www.oecd.org/pisa/keyfindings/pisa-2012-results-overview.pdf
https://www.oecd.org/pisa/keyfindings/PISA-2012-results-US.pdf
http://www.corestandards.org/about-the-standards/
https://www.aei.org/wp-content/uploads/2012/08/-solving-americas-mathematics-education-problem_085301336532.pdf
http://www2.ed.gov/about/bdscomm/list/mathpanel/report/final-report.pdf
http://mtoliveboe.org/cmsAdmin/uploads/blackwell-theories-of-intelligence-child-dev-2007.pdf
http://mdk12.msde.maryland.gov/instruction/curriculum/mathematics/cognitive_levels.html








